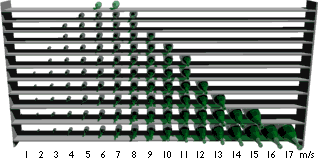
Mean (Average) Power of the Wind Balancing the Power Distribution The reason why we care about wind speeds is their energy content, just like with the bottles on the previous page: We cared about their content in terms of volume. Now, the volume of a bottle varies with the cube of the size, just like wind power varies with the cube of the wind speed. Let us take the Weibull distribution of wind speeds, and for each speed we place a bottle on a shelf each time we have a 1 per cent probability of getting that wind speed. The size of each bottle corresponds to the wind speed, so the weight of each bottle corresponds to the amount of energy in the wind. To the right, at 17 m/s we have some really heavy bottles, which weigh almost 5000 times as much as the bottles at 1 m/s. (At 1 m/s the wind has a power of 0.61 W/m2. At 17 m/s its power is 3009 W/m2). Finding the wind speed at which we get the mean of the power distribution is equivalent to balancing the bookshelves. (Remember how we did the balancing act on the Weibull distribution page?). In this case, as you can see, although high winds are rare, they weigh in with a lot of energy. So, in this case with an average wind speed of 7 m/s, the power weighted average of wind speeds is 8.7 m/s. At that wind speed the power of the wind is 402 W/m2, which is almost twice as much as we figured out in our naive calculation on the top of the previous page. On the next pages we will use a more convenient method of finding the power in the wind than hauling bottles around...
	| Back | Home | Forward | © Copyright 2000 Soren Krohn. All rights reserved. Updated 9 September 2000 http://www.windpower.org/tour/wres/shelves.htm