Annual Energy Output from a Wind Turbine

We are now ready to calculate the relationship between average wind speeds and annual energy output from a wind turbine.
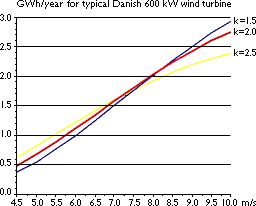
To draw the graph to the right, we have used the power calculator on the previous page, and the power curve from the default example 600 kW wind turbine. We have used a standard atmosphere with an air density of 1.225 kg/m³.
For each of the Weibull parameters 1.5, 2.0, and 2.5 we have calculated the annual energy output for different average wind speeds at turbine hub height.
As you can see, output may vary up to 50 per cent depending on the shape parameter at a low average wind speed of 4.5 m/s, while it may vary some 30 per cent at a very high average wind speed of 10 m/s at hub height.

Output varies almost with the cube of the wind speed
Now, let us look at the red curve with k=2, which is the curve normally shown by manufacturers:
With an average wind speed of 4.5 m/s at hub height the machine will generate about 0.5 GWh per year, i.e. 500,000 kWh per year. With an average wind speed of 9 metres per second it will generate 2.4 GWh/year = 2,400,000 kWh per year. Thus, doubling the average wind speed has increased energy output 4.8 times.
If we had compared 5 and 10 metres per second instead, we would have obtained almost exactly 4 times as much energy output.
The reason why we do not obtain exactly the same results in the two cases, is that the efficiency of the wind turbine varies with the wind speeds, as described by the power curve. Note, that the uncertainty that applies to the power curve also applies to the result above.
You may refine your calculations by accounting for the fact that e.g. in temperate climates the wind tends to be stronger in winter than in summer, and stronger during the daytime than at night.

The Capacity Factor
Another way of stating the annual energy output from a wind turbine is to look at the capacity factor for the turbine in its particular location. By capacity factor we mean its actual annual energy output divided by the theoretical maximum output, if the machine were running at its rated (maximum) power during all of the 8766 hours of the year.
Example: If a 600 kW turbine produces 1.5 million kWh in a year, its capacity factor is = 1500000 : ( 365.25 * 24 * 600 ) = 1500000 : 5259600 = 0.285 = 28.5 per cent.
Capacity factors may theoretically vary from 0 to 100 per cent, but in practice they will usually range from 20 to 70 per cent, and mostly be around 25-30 per cent.

The Capacity Factor Paradox
Although one would generally prefer to have a large capacity factor, it may not always be an economic advantage. This is often confusing to people used to conventional or nuclear technology.
In a very windy location, for instance, it may be an advantage to use a larger generator with the same rotor diameter (or a smaller rotor diameter for a given generator size). This would tend to lower the capacity factor (using less of the capacity of a relatively larger generator), but it may mean a substantially larger annual production, as you can verify using the Power calculator on this web site.
Whether it is worthwhile to go for a lower capacity factor with a relatively larger generator, depends both on wind conditions, and on the price of the different turbine models of course.
Another way of looking at the capacity factor paradox is to say, that to a certain extent you may have a choice between a relatively stable power output (close to the design limit of the generator) with a high capacity factor - or a high energy output (which will fluctuate) with a low capacity factor.